3D Hyperbolic Tiling and Horosphere Cross Section

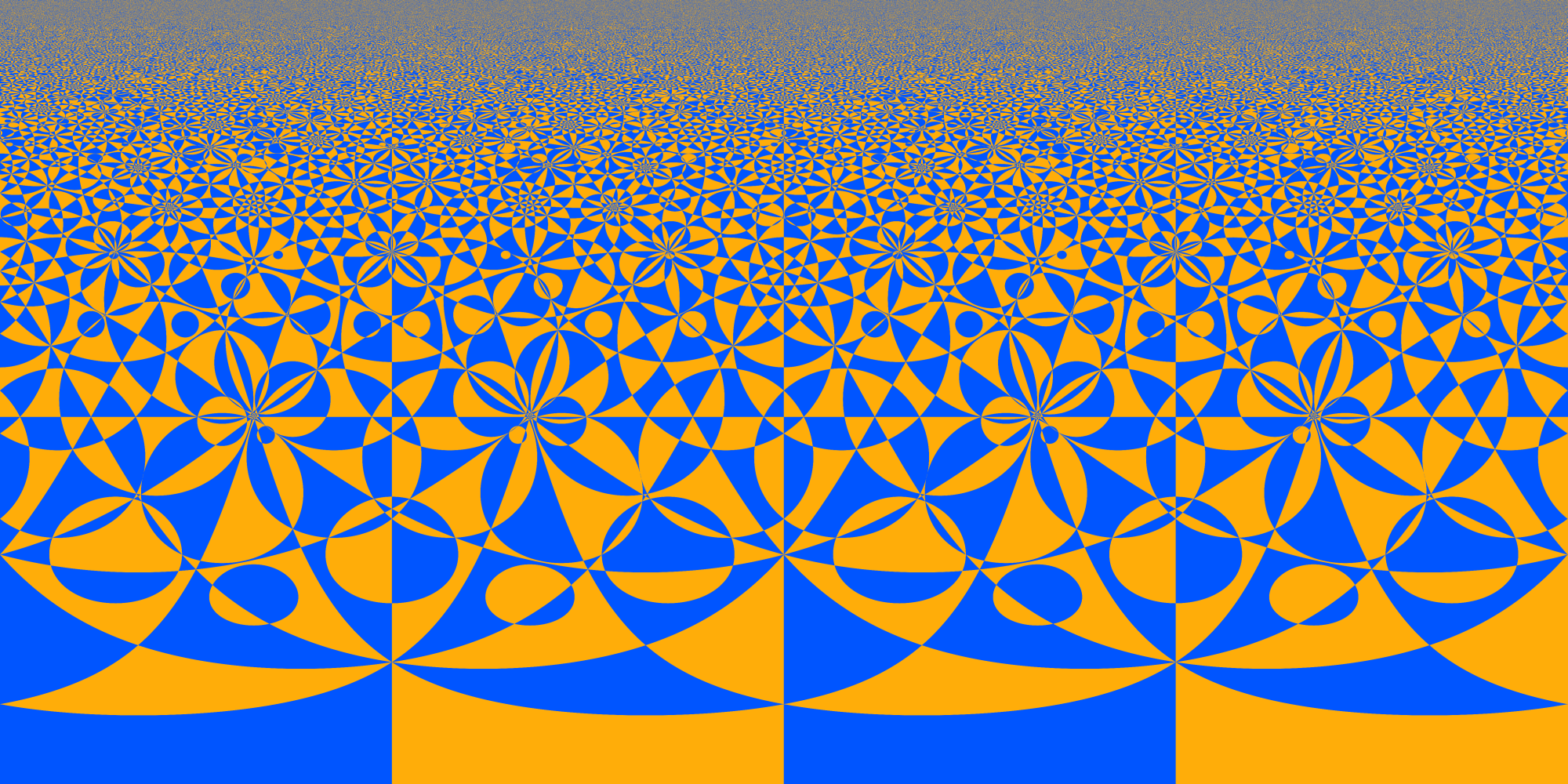
The images of two dimensional hyperbolic tilings are familiar and were popularized by M.C.Escher Circle Limit woodcuts. Tilings of 3 dimensional hyperbolic space are much harder to visualize because viewer has to be inside of the hyperbolic space to be able to see the tiling completely. We try to display 3D tilings as a two dimensional cross sections with horospheres. The intrinsic geometry of horosphere is euclidean plane therefore horosphere can naturally be displayed in two dimensions without distortions. Such a horosphere cross sections display interesting properties. It is infinite and is somewhat similar everywhere but non repeating. The apparent size of individual tiles sections remains constant in contrast to 2d tiling where tiles size rapidly tends to zero when tiles approach the hyperbolic plane horizon. We will provide few examples of such a tilings and several animations.