

Visual representation of tiling of 3D hyperbolic space attracted very little attention compare to tilings of hyperbolic plane, which were popularized by M.C.Escher circle limit woodcuts. Although there is a lot of activity on theoretical side of the problem starting from work of H.Poincare on Kleinian groups and continuing with breakthrough of W.Thurston in the development of low dimensional topology and G.Perelman's proof of Poincare conjecture.
The book "Indra's Pearl" have popularized visualization of 2D limit set of Kleinian groups, which is located at the infinity of hyperbolic space. In this talk we present our attempts to build and visualize actual 3D tilings. We study tilings with symmetry group generated by reflections in the faces of Coxeter polyhedron, which also is the fundamental polyhedron of the group.
Online location bulatov.org/math/1101/
PDF version(6.5MB) in one file.
HTML version. It requires browser supporting SVG and MathML, such as FireFox. It works like PowerPoint presentation and has over 130 pages.
Here are few selected images.
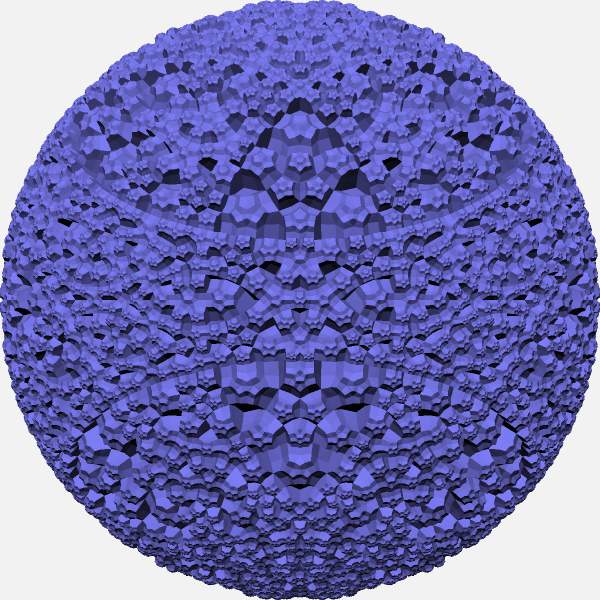 Tiling of hyperbolic space with right angled dodecahedra.
Tiling of hyperbolic space with right angled dodecahedra.
 Partial tiling with right angled dodecahedra.
Partial tiling with right angled dodecahedra.
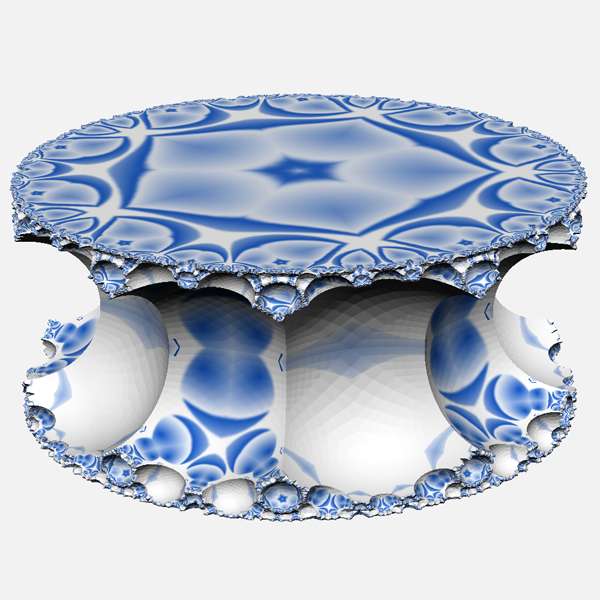 Partial tiling shown in cylinder model.
Partial tiling shown in cylinder model.
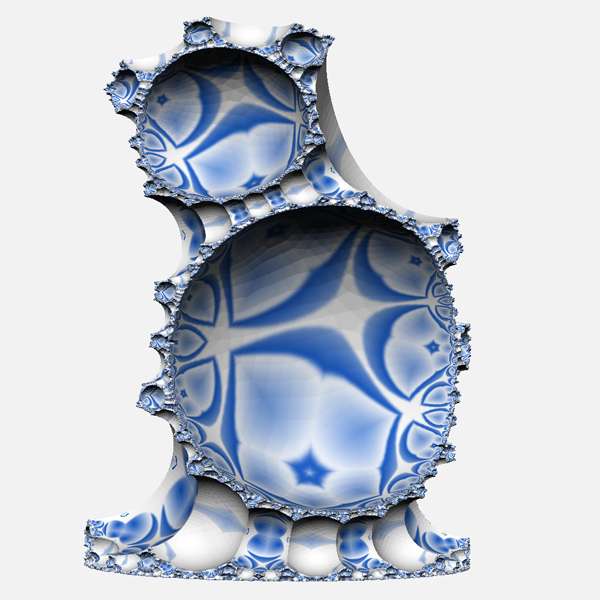 Another view of the same partial tiling.
Another view of the same partial tiling.
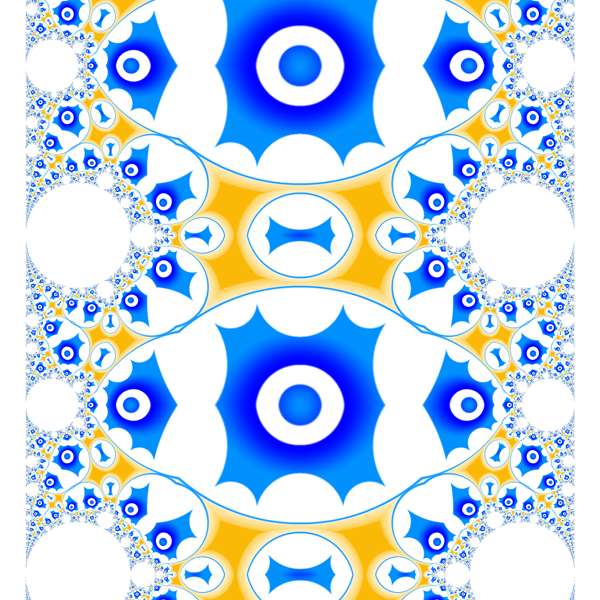 Tiling on the infinity of the hyperbolic space.
Tiling on the infinity of the hyperbolic space.